Решите неравенство: (x^3+x^2-16x+16)/(x+1)<=0.

\[\frac{x^{3} + x^{2} - 16x + 16}{x + 1} \leq 0\]

\[\frac{x^{2}(x + 1) - 16 \cdot (x - 1)}{x + 1} \leq 0\ \ \rightarrow\]

\[\rightarrow не\ раскладывается\ \]

\[на\ множители.\]

\[\frac{x^{3} + x^{2} - 16x - 16}{x + 1} \leq 0\]

\[\frac{x^{2}(x + 1) - 16 \cdot (x + 1)}{x + 1} \leq 0\]

\[\frac{(x + 1)(x^{2} - 16)}{x + 1} \leq 0\]

\[\frac{(x + 1)(x - 4)(x + 4)}{x + 1} \leq 0\]

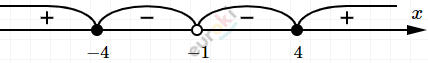
\[Ответ:\lbrack - 4; - 1) \cup ( - 1;4\rbrack.\]