Алгебра
Английский
Биология
География
Геометрия
История
Русский
Обществознание
Физика
Химия
Информатика
Литература
МатематикаРешебник по алгебре 9 класс Мерзляк Задание 810
Задание 810
\[\boxed{\mathbf{810\ (810).\ }Еуроки\ - \ ДЗ\ без\ мороки}\]
\[S = \frac{a_{1} + a_{n}}{2} \cdot n = \frac{10 + 99}{2} \cdot 90 =\]
\[= 109 \cdot 45 = 4905 - все\]
\[\ двузначные\ числа.\]
\[Кратны\ трем:\ \ \ 12,\ 15,\ 18,\ \ldots,\]
\[\ 99,\ldots \Longrightarrow \text{\ \ }d = 3.\]
\[a_{n} = a_{1} + d(n - 1)\ \]
\[99 = 12 + 3 \cdot (n - 1)\ \]
\[99 = 12 + 3n - 3\]
\[3n = 90\]
\[n = 30.\ \ \]
\[Тогда:\]
\[S^{3} = \frac{a_{1} + a_{n}}{2} \cdot n =\]
\[= \frac{12 + 99}{2} \cdot 30 = 115 \cdot 15 =\]
\[= 1665.\]
\[Кратны\ пяти:\ \ \ \ 10\ ,15,\ \ldots,\ 95,\]
\[\ldots \Longrightarrow \text{\ \ }d = 5.\]
\[a_{n} = a_{1} + d(n - 1)\]
\[95 = 10 + 5 \cdot (n - 1)\ \]
\[95 = 10 + 5n - 5\]
\[5n = 90\]
\[n = 18.\ \ \]
\[Тогда:\]
\[S^{5} = \frac{a_{1} + a_{n}}{2} \cdot n =\]
\[= \frac{10 + 95}{2} \cdot 18 = 105 \cdot 9 = 945.\]
\[Ни\ на\ 3,\ ни\ на\ 5:\ \ 3 \cdot 5 = 15.\]
\[Делятся\ на\ 15:15,\ 30,\ 45,\ \ldots,\]
\[90 \Longrightarrow \text{\ \ }d = 15.\]
\[a_{n} = a_{1} + d(n - 1)\ \]
\[90 = 15 + 15 \cdot (n - 1)\text{\ \ }\]
\[90 = 15 + 15n - 15\]
\[15n = 90\ \]
\[\ n = 6\]
\[S^{15} = \frac{a_{1} + a_{n}}{2} \cdot n =\]
\[= \frac{15 + 90}{2} \cdot 6 = 105 \cdot 3 = 315.\]
\[Тогда\ искомая\ сумма:\]
\[S = S - S^{3} - S^{5} + S^{15} = 4905 -\]
\[- 1665 - 945 + 315 = 2610.\]
\[Ответ:2610.\]
\[\boxed{\mathbf{810.\ }Еуроки\ - \ ДЗ\ без\ мороки}\]
\[1)\ y = \frac{4}{\sqrt{x^{2} + 3x - 10}} + \frac{1}{3x - 9}\]
\[\left\{ \begin{matrix} x^{2} + 3x - 10 > 0 \\ 3x - 9 \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ }\]
\[\left\{ \begin{matrix} (x + 5)(x - 2) > 0 \\ x \neq 3\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
\[Ответ:\]
\[x \in ( - \infty; - 5) \cup (2;3) \cup (3; + \infty).\]
\[2)\ y = \frac{6}{\sqrt{12 + x - x²}} - \frac{2}{x² - 4}\]
\[\left\{ \begin{matrix} 12 + x - x^{2} > 0 \\ x^{2} - 4 \neq 0\ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ }\]
\[\left\{ \begin{matrix} x^{2} - x - 12 < 0 \\ x \neq \pm 2\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ }\]
\[\left\{ \begin{matrix} (x - 4)(x + 3) < 0 \\ x \neq \pm 2\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
\[Ответ:\]
\[x \in ( - 3; - 2) \cup ( - 2;2) \cup (2;4).\]
\[3)\ y = \sqrt{49 - x^{2}} + \frac{1}{\sqrt{x^{2} + 3x - 4}}\]
\[\left\{ \begin{matrix} 49 - x^{2} \geq 0\ \ \ \ \ \ \\ x^{2} + 3x - 4 > 0 \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ }\]
\[\ \left\{ \begin{matrix} (7 - x)(7 + x) \geq 0 \\ (x + 4)(x - 1) > 0 \\ \end{matrix} \right.\ \]
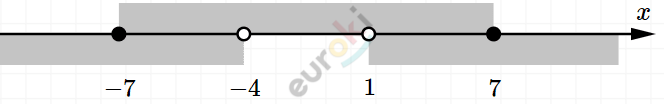
\[Ответ:x \in \lbrack - 7;\ - 4) \cup (1;7\rbrack.\]
