Решебник по алгебре 8 класс Макарычев ФГОС Задание 478

 

\[\boxed{\text{478\ (478).}\text{\ }\text{Еуроки\ -\ ДЗ\ без\ мороки}}\]

\[Разложим\ подкоренные\ \]

\[выражения\ на\ множители.\]

\[\textbf{а)}\ 15\sqrt{20} \cdot 0,1\sqrt{45} =\]

\[= 15 \cdot \sqrt{4} \cdot \sqrt{5} \cdot 0,1 \cdot \sqrt{9} \cdot \sqrt{5} =\]

\[= 15 \cdot 0,1 \cdot 2\sqrt{5} \cdot 3\sqrt{5} =\]

\[= 1,5 \cdot 6 \cdot 5 = 45\]

\[\textbf{б)}\ 0,3\sqrt{10} \cdot 0,2\sqrt{15} \cdot 0,5\sqrt{6} =\]

\[= 0,3 \cdot 0,2 \cdot 0,5 \cdot \sqrt{10 \cdot 15 \cdot 6} =\]

\[= 0,03\sqrt{900} = 0,03 \cdot 30 = 0,9\]

\[\textbf{в)}\ \frac{8\sqrt{5}}{0,4\sqrt{0,2}} = \frac{8\sqrt{5}}{\frac{4}{10} \cdot \sqrt{\frac{1}{5}}} =\]

\[= \frac{8 \cdot 10}{4} \cdot \sqrt{5} \cdot \sqrt{5} = \frac{80 \cdot \sqrt{25}}{4} =\]

\[= 20 \cdot 5 = 100\]

\[\textbf{г)}\ \frac{\sqrt{0,48}}{5\sqrt{12}} = \frac{1}{5} \cdot \sqrt{\frac{0,48}{12}} =\]

\[= \frac{1}{5} \cdot \sqrt{\frac{48}{1200}} = \frac{1}{5} \cdot \sqrt{\frac{4}{100}} =\]

\[= \frac{1}{5} \cdot \frac{2}{10} = \frac{2}{50} = 0,04\]

\[\boxed{\text{478.}\text{\ }\text{еуроки}\text{-}\text{ответы}\text{\ }\text{на}\text{\ }\text{пятёрку}}\]

Пояснение.

При любом значении x верно равенство:

\[\sqrt{x^{2}} = |x|.\]

Модулем числа a называется само число a, если a>=0, или (-a), если a<0:

\[|a| = a;при\ a \geq 0;\]

\[|a| = - a;при\ a < 0.\]

Модуль числа всегда или положительное число, или равен 0.

Решение.

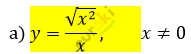
\[\left\{ \begin{matrix} y = \frac{x}{x},\ \ x > 0\ \ \\ y = \frac{x}{- x},\ \ \ x < 0\ \ \ \ \\ \end{matrix} \right.\ \]

\[\left\{ \begin{matrix} y = - \frac{2x}{x} = - 2,\ \ \ \ x > 0 \\ y = - \frac{2x}{- x} = 2,\ \ \ \ x < 0 \\ \end{matrix} \right.\ \]

\[\left\{ \begin{matrix} y = x \cdot x = x^{2},\ \ \ \ \ \ \ \ \ \ x > 0 \\ y = - x \cdot x = - x^{2},\ \ \ \ x < 0 \\ \end{matrix} \right.\ \ \]

\[\left\{ \begin{matrix} y = - x \cdot x = - x^{2},\ \ \ \ x > 0 \\ y = - ( - x) \cdot x = x^{2},\ \ \ \ x < 0 \\ \end{matrix} \right.\ \]