Решебник по алгебре и начала математического анализа 11 класс Алимов Задание 481

\[\boxed{\mathbf{481.}}\]

\[1)\cos{135{^\circ}} = \cos(90{^\circ} + 45{^\circ}) =\]

\[= 0 \bullet \frac{\sqrt{2}}{2} - 1 \bullet \frac{\sqrt{2}}{2} = - \frac{\sqrt{2}}{2}\]

\[2)\cos{120{^\circ}} = \cos(60{^\circ} + 60{^\circ}) =\]

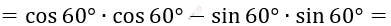
\[= \frac{1}{2} \bullet \frac{1}{2} - \frac{\sqrt{3}}{2} \bullet \frac{\sqrt{3}}{2} = \frac{1}{4} - \frac{3}{4} =\]

\[= - \frac{2}{4} = - \frac{1}{2}\]

\[3)\cos{150{^\circ}} = \cos(90{^\circ} + 60{^\circ}) =\]

\[= 0 \bullet \frac{1}{2} - 1 \bullet \frac{\sqrt{3}}{2} = - \frac{\sqrt{3}}{2}\]

\[4)\cos{240{^\circ}} = \cos(180{^\circ} + 60{^\circ}) =\]

\[= - 1 \bullet \frac{1}{2} - 0 \bullet \frac{\sqrt{3}}{2} = - \frac{1}{2}\]