Решебник по алгебре и начала математического анализа 11 класс Алимов Задание 351

\[\boxed{\mathbf{351}\mathbf{.}}\]

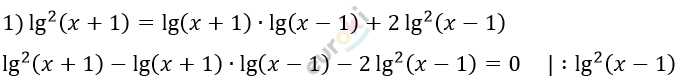
\[\left( \frac{\lg(x + 1)}{\lg(x - 1)} \right)^{2} - \frac{\lg(x + 1)}{\lg(x - 1)} - 2 = 0\]

\[Пусть\ y = \frac{\lg(x + 1)}{\lg(x - 1)}:\]

\[y^{2} - y - 2 = 0\]

\[D = 1^{2} + 4 \bullet 2 = 1 + 8 = 9\]

\[y_{1} = \frac{1 - 3}{2} = - 1;\text{\ \ }\]

\[y_{2} = \frac{1 + 3}{2} = 2.\]

\[1)\ \frac{\lg(x + 1)}{\lg(x - 1)} = - 1\]

\[\lg(x + 1) = - \lg(x - 1)\]

\[\lg(x + 1) = \lg(x - 1)^{- 1}\]

\[x + 1 = (x - 1)^{- 1}\]

\[x + 1 = \frac{1}{x - 1}\]

\[(x + 1)(x - 1) = 1\]

\[x^{2} - 1 = 1\]

\[x^{2} = 2\]

\[x = \pm \sqrt{2}.\]

\[2)\ \frac{\lg(x + 1)}{\lg(x - 1)} = 2\]

\[\lg(x + 1) = 2\lg(x - 1)\]

\[\lg(x + 1) = \lg(x - 1)^{2}\]

\[x + 1 = (x - 1)^{2}\]

\[x + 1 = x^{2} - 2x + 1\]

\[x^{2} - 3x = 0\]

\[x(x - 3) = 0\]

\[x_{1} = 0;\ x_{2} = 3.\]

\[имеет\ смысл\ при:\]

\[x + 1 > 0 \Longrightarrow \ x > - 1;\]

\[x - 1 > 0 \Longrightarrow x > 1.\]

\[Ответ:\ \ x_{1} = \sqrt{2};\ \ x_{2} = 3.\]

\[2)\ 2\log_{5}(4 - x) \bullet \log_{2x}(4 - x) =\]

\[= 3\log_{5}(4 - x) - \log_{5}(2x)\]

\[Пусть\ y = \frac{\log_{5}(4 - x)}{\log_{5}(2x)}:\]

\[2y^{2} - 3y + 1 = 0\]

\[D = 3^{2} - 4 \bullet 2 = 9 - 8 = 1\]

\[y_{1} = \frac{3 - 1}{2 \bullet 2} = \frac{2}{4} = \frac{1}{2};\]

\[y_{2} = \frac{3 + 1}{2 \bullet 2} = \frac{4}{4} = 1.\]

\[1)\ \frac{\log_{5}(4 - x)}{\log_{5}(2x)} = \frac{1}{2}\]

\[2\log_{5}(4 - x) = \log_{5}(2x)\]

\[\log_{5}(4 - x)^{2} = \log_{5}(2x)\]

\[(4 - x)^{2} = 2x\]

\[16 - 8x + x^{2} = 2x\]

\[x^{2} - 10x + 16 = 0\]

\[D = 10^{2} - 4 \bullet 16 =\]

\[= 100 - 64 = 36\]

\[x_{1} = \frac{10 - 6}{2} = 2;\text{\ \ }\]

\[x_{2} = \frac{10 + 6}{2} = 8.\]

\[2)\ \frac{\log_{5}(4 - x)}{\log_{5}(2x)} = 1\]

\[\log_{5}(4 - x) = \log_{5}(2x)\]

\[4 - x = 2x\]

\[3x = 4\]

\[\ x = \frac{4}{3}\]

\[x = 1\frac{1}{3}.\]

\[имеет\ смысл\ при:\]

\[4 - x > 0 \Longrightarrow x < 4;\]

\[2x > 0 \Longrightarrow x > 0.\]

\[Ответ:\ \ x_{1} = 2;\ \ x_{2} = 1\frac{1}{3}.\]