Решите систему уравнений: x^3-y^3=26; x^2+xy+y^2=13.

Ответ:

\[\left\{ \begin{matrix} x^{3} - y^{3} = 26\ \ \ \ \ \ \ \ \ \ \\ x^{2} + xy + y^{2} = 13 \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ }\]

\[\left\{ \begin{matrix} (x - y)\left( x^{2} + xy + y^{2} \right) = 26 \\ x^{2} + xy + y^{2} = 13\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ \ }\]

\[\left\{ \begin{matrix} 13 \cdot (x - y) = 26\ \ \\ x² + xy + y² = 13 \\ \end{matrix} \right.\ \]

\[\left\{ \begin{matrix} x - y = 2\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ x^{2} + xy + y^{2} = 13 \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ \ \ \ \ }\]

\[\left\{ \begin{matrix} y = x - 2\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ x² + x(x - 2) + (x - 2)^{2} = 13 \\ \end{matrix} \right.\ \]

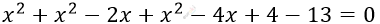
\[3x² - 6x - 9 = 0\ \ \ |\ :3\]

\[x^{2} - 2x - 3 = 0\]

\[x_{1} + x_{2} = 2,\ \ \ x_{1} \cdot x_{2} = - 3\]

\[x_{1} = 3,\ \ x_{2} = - 1\]

\[\left\{ \begin{matrix} x = 3 \\ y = 1 \\ \end{matrix} \right.\ \ \ \ \ \ \ \ \ \ или\ \ \ \ \left\{ \begin{matrix} x = - 1 \\ y = - 3 \\ \end{matrix} \right.\ \]

\[Ответ:( - 1;\ - 3);(3;1).\]