Решите неравенство: 8x/(x+5)-3*корень из (8x/(x+5))-4>=0.

\[\frac{8x}{x + 5} - 3\sqrt{\frac{8x}{x + 5}} - 4 \geq 0;\ \ \ \]

\[\ t = \sqrt{\frac{8x}{x + 5}}\ \]

\[t^{2} - 3t - 4 \geq 0\]

\[(t - 4)(t + 1) \geq 0\]

\[t \leq 1;\ \ \ \ \ t \geq 4.\]

\[1)\ \sqrt{\frac{8x}{x + 5}} \leq - 1\]

\[\sqrt{\frac{8x}{x + 5}} \geq 0 \Longrightarrow нет\ решения.\]

\[2)\sqrt{\frac{8x}{x + 5}} \geq 4\]

\[\frac{8x}{x + 5} \geq 16\]

\[\frac{8x - 16 \cdot (x + 5)}{x + 5} \geq 0\]

\[\frac{8x - 16x - 80}{x + 5} \geq 0\]

\[\frac{- 8x - 80}{x + 5} \geq 0\]

\[\frac{- 8 \cdot (x + 10)}{x + 5} \geq 0\]

\[\frac{x + 10}{x + 5} \leq 0\ \]

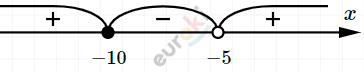
\[Ответ:\lbrack - 10; - 5).\]