Решебник по алгебре 9 класс Макарычев Задание 443

 

\[\boxed{\text{443\ (443).}\text{\ }\text{Еуроки\ -\ ДЗ\ без\ мороки}}\]

Пояснение.

Решение.

\[\textbf{а)}\ \left\{ \begin{matrix} x - y = 5 \\ \frac{1}{x} + \frac{1}{y} = \frac{1}{6} \\ \end{matrix} \right.\ \Longrightarrow\]

\[\Longrightarrow \left\{ \begin{matrix} x = y + 5\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{1^{\backslash 6y}}{y + 5} + \frac{1^{\backslash y + 5}}{y} = \frac{1^{\backslash y(y + 5)}}{6} \\ \end{matrix} \right.\ \]

\[\Longrightarrow \left\{ \begin{matrix} x = y + 5\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{6y + 6 \cdot (y + 5) - y(y + 5)}{6y(y + 5)} = 0 \\ \end{matrix} \right.\ \]

\[6y + 6y + 30 - y^{2} - 5y = 0\]

\[y^{2} - 7y - 30 = 0\]

\[D = 7^{2} + 4 \cdot 30 = 169\]

\[y_{1,2} = \frac{7 \pm 13}{2} = 10; - 3.\]

\[1)\ y_{1} = 10;\ \ x_{1} = 15;\]

\[2)\ y_{2} = - 3;\ \ x_{2} = 2.\ \ \]

\[\textbf{б)}\ \left\{ \begin{matrix} x + y = 6 \\ \frac{1}{x} - \frac{1}{y} = \frac{1}{4} \\ \end{matrix} \right.\ \Longrightarrow\]

\[\Longrightarrow \left\{ \begin{matrix} y = 6 - x\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{1^{\backslash 6 - x}}{x} - \frac{1^{\backslash 4x}}{6 - x} = \frac{1^{\backslash x(6 - x)}}{4} \\ \end{matrix} \right.\ \]

\[\Longrightarrow \left\{ \begin{matrix} y = 6 - x\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{4 \cdot (6 - x) - 4x - x(6 - x)}{4x(6 - x)} = 0 \\ \end{matrix} \right.\ \]

\[\Longrightarrow \left\{ \begin{matrix} y = 6 - x\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{24 - 4x - 4x - 6x + x^{2}}{4x(6 - x)} = 0 \\ \end{matrix} \right.\ \]

\[x^{2} - 14x + 24 = 0\]

\[D_{1} = 49 - 24 = 25\]

\[x_{1} = 7 - 2 = 2;\ \]

\[\ x_{2} = 7 + 5 = 12.\]

\[1)\ x_{1} = 2;\ \ y_{1} = 4;\]

\[2)\ x_{2} = 12;\ \ y_{2} = - 6.\]

\[\textbf{в)}\ \left\{ \begin{matrix} 3x + y = 1\ \ \ \\ \frac{1}{x} + \frac{1}{y} = - 2,5 \\ \end{matrix} \right.\ \Longrightarrow\]

\[\Longrightarrow \left\{ \begin{matrix} y = 1 - 3x\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{1^{\backslash 1 - 3x}}{x} + \frac{1^{\backslash x}}{1 - 3x} = - {2,5}^{\backslash x(1 - 3x)} \\ \end{matrix} \right.\ \]

\[\Longrightarrow \left\{ \begin{matrix} y = 1 - 3x\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{2 - 6x + 2x + 5x - 15x^{2}}{x(1 - 3x)} = 0 \\ \end{matrix} \right.\ \]

\[- 15x^{2} + x + 2 = 0\]

\[15x^{2} - x - 2 = 0\]

\[D = 1 + 120 = 121\]

\[x_{1,2} = - \frac{1}{3};\ \ \ \frac{2}{5}.\]

\[1)\ x_{1} = - \frac{1}{3};\ \ y_{1} = 2;\]

\[2)\ x_{2} = \frac{2}{5};\ \ y_{2} = - \frac{1}{5}.\]

\[\textbf{г)}\ \left\{ \begin{matrix} \frac{1^{\backslash 3x}}{y} - \frac{1^{\backslash 3y}}{x} = \frac{1^{\backslash xy}}{3}\text{\ \ } \\ x - 2y = 3\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \Longrightarrow\]

\[\Longrightarrow \left\{ \begin{matrix} x = 2y + 3\ \ \ \ \ \ \ \ \ \ \ \ \\ \frac{3x - 3y - xy}{3xy} = 0 \\ \end{matrix} \right.\ \Longrightarrow\]

\[2y^{2} - y - 6 = 0\]

\[D = 1 + 4 \cdot 2 \cdot 6 = 49\]

\[y_{1,2} = \frac{1 \pm 7}{4} = 2;\ - 1,5.\]

\[1)\ y_{1} = 2;\ \ \ \ x_{1} = 6;\]

\[2)\ y_{2} = - 1,5;\ \ \ x_{2} = - 1.\]

\[Ответ:а)\ (15;10);(2;\ - 3);\ \ \]

\[\textbf{б)}\ (2;4);\ \ (12;\ - 6);\]

\[\textbf{в)}\ \left( - \frac{1}{3};2 \right);\ \ \left( \frac{2}{5};\ - \frac{1}{5} \right);\ \]

\[\ г)\ (6;2);( - 1;\ - 1,5).\]

\(\boxed{\text{443.}\text{\ }\text{ОК\ ГДЗ\ -\ домашка\ на\ 5}}\)

\[\textbf{а)} - \sqrt{6}.\]

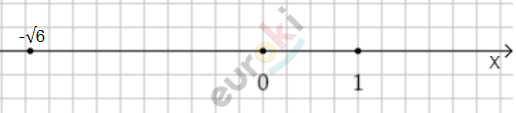
\[\textbf{б)}\ \sqrt{2} \approx 1,41;\ \ \sqrt{3} \approx 1,73\]

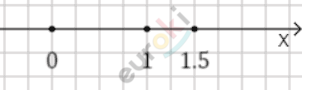
\[Число:1,5.\]

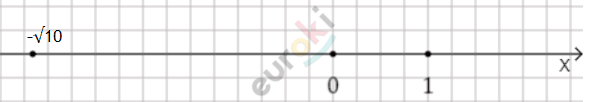
\[\textbf{в)} - \sqrt{10}.\]

\[\textbf{г)}\ \frac{1}{3} \rightarrow \frac{1}{9} = \frac{4}{36};\ \frac{1}{2} \rightarrow \frac{1}{4} = \frac{9}{36}\]

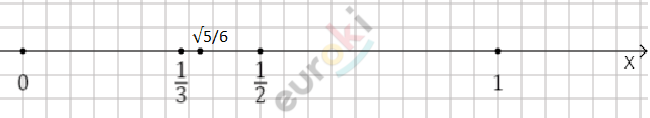
\[Число:\ \frac{\sqrt{5}}{6}\]