Алгебра
Английский
Биология
География
Геометрия
История
Русский
Обществознание
Физика
Химия
Информатика
Литература
МатематикаРешебник по алгебре 9 класс Макарычев Задание 336
Задание 336
\[\boxed{\text{336\ (}\text{н}\text{).}\text{\ }\text{Еуроки\ -\ ДЗ\ без\ мороки}}\]
\[\textbf{а)}\ \frac{x - 1}{x - 3} \geq 0\]
\[\left\{ \begin{matrix} (x - 1)(x - 3) \geq 0 \\ x \neq 3\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \ \]
\[x \in ( - \infty;1\rbrack \cup (3; + \infty).\]
\[\textbf{б)}\ \frac{x + 6}{x - 5} \leq 0\]
\[\left\{ \begin{matrix} (x + 6)(x - 5) \leq 0 \\ x \neq 5\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
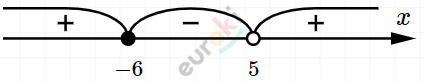
\[\ x \in \lbrack - 6;5).\]
\[\textbf{в)}\ \frac{2 - x}{x} \geq 0\]
\[\left\{ \begin{matrix} x(2 - x) \geq 0 \\ x \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \Longrightarrow \left\{ \begin{matrix} x(x - 2) \leq 0 \\ x \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
\[\ x \in (0;2\rbrack.\]
\[\textbf{г)}\ \frac{3 - 2x}{x - 1} \leq 0\]
\[\left\{ \begin{matrix} (3 - 2x)(x - 1) \leq 0 \\ x \neq 1\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \Longrightarrow \ \]
\[\Longrightarrow \left\{ \begin{matrix} 2 \cdot (x - 1)(x - 1,5) \geq 0 \\ x \neq 1\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
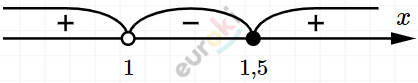
\[x \in ( - \infty;1) \cup \lbrack 1,5; + \infty).\]
\[\textbf{д)}\ \frac{7x - 2}{1 - x} \geq 0\]
\[\left\{ \begin{matrix} (7x - 2)(1 - x) \geq 0 \\ 1 - x \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \Longrightarrow\]
\[\Longrightarrow \left\{ \begin{matrix} 7 \cdot \left( x - \frac{2}{7} \right)(x - 1) \leq 0 \\ x \neq 1\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
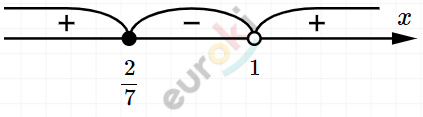
\[x \in \left\lbrack \frac{2}{7};1 \right).\]
\[\textbf{е)}\ \frac{1 - 11x}{2x - 3} \leq 0\]
\[\left\{ \begin{matrix} (1 - 11x)(2x - 3) \leq 0 \\ 2x - 3 \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \Longrightarrow\]
\[\Longrightarrow \left\{ \begin{matrix} 11 \cdot 2 \cdot \left( x - \frac{1}{11} \right)(x - 1,5) \geq 0 \\ x \neq 1,5\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
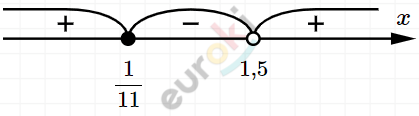
\[x \in \left( - \infty;\frac{1}{11} \right\rbrack \cup (1,5; + \infty).\]
\[\boxed{\text{336\ (}\text{c}\text{).}\text{\ }\text{Еуроки\ -\ ДЗ\ без\ мороки}}\]
\[\textbf{а)}\ \frac{x - 1}{x - 3} \geq 0\]
\[\left\{ \begin{matrix} (x - 1)(x - 3) \geq 0 \\ x \neq 3\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
\[\ x \in ( - \infty;1\rbrack \cup (3; + \infty).\]
\[\textbf{б)}\ \frac{x + 6}{x - 5} \leq 0\]
\[\left\{ \begin{matrix} (x + 6)(x - 5) \leq 0 \\ x \neq 5\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ }\]
\[x \in \lbrack - 6;5).\]
\[\textbf{в)}\ \frac{2 - x}{x} \geq 0\]
\[\left\{ \begin{matrix} x(2 - x) \geq 0 \\ x \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \Longrightarrow \left\{ \begin{matrix} x(x - 2) \leq 0 \\ x \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ }\]
\[x \in (0;2\rbrack.\]
\[\textbf{г)}\ \frac{3 - 2x}{x - 1} \leq 0\]
\[\left\{ \begin{matrix} (3 - 2x)(x - 1) \leq 0 \\ x \neq 1\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \Longrightarrow\]
\[\Longrightarrow \ \left\{ \begin{matrix} 2 \cdot (x - 1)(x - 1,5) \geq 0 \\ x \neq 1\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \]
\[x \in ( - \infty;1) \cup \lbrack 1,5; + \infty).\]
\[\boxed{\text{336.}\text{\ }\text{ОК\ ГДЗ\ -\ домашка\ на\ 5}}\]
\[\textbf{а)}\ 2 \cdot \left( x^{2} + \frac{1}{x^{2}} \right) - \left( x + \frac{1}{x} \right) = 2\]
\[Пусть\ \ t = x + \frac{1}{x};\ \ \]
\[t^{2} = \left( x + \frac{1}{x} \right)^{2} = x^{2} + 2 + \frac{1}{x^{2}};\ \]
\[x^{2} + \frac{1}{x^{2}} = t^{2} - 2:\]
\[2 \cdot \left( t^{2} - 2 \right) - t = 2\]
\[2t^{2} - 4 - t - 2 = 0\]
\[2t^{2} - t - 6 = 0\]
\[D = 1 + 4 \cdot 2 \cdot 6 = 49\]
\[t_{1,2} = \frac{1 \pm 7}{4} = 2;\ - \frac{3}{2}\text{.\ }\]
\[1)\ x + \frac{1}{x} = 2\]
\[x^{2} - 2x + 1 = 0\ \ \]
\[(x - 1)^{2} = 0\ \ \]
\[x = 1.\]
\[2)\ x + \frac{1}{x} = - \frac{3}{2}\text{\ \ }\]
\[2x^{2} + 3x + 2 = 0\]
\[D = 9 - 4 \cdot 2 \cdot 2 < 0 \Longrightarrow\]
\[\Longrightarrow корней\ нет.\]
\[Ответ:x = 1.\]
\[\textbf{б)}\ \ 9x^{2} - 18x + \frac{9}{x^{2}} - \frac{18}{x} = 22\]
\[9 \cdot \left( x^{2} + \frac{1}{x^{2}} \right) - 18 \cdot \left( x + \frac{1}{x} \right) =\]
\[= 22.\]
\[Пусть\ \ \ t = x + \frac{1}{x};\ \ \]
\[x^{2} + \frac{1}{x^{2}} = t^{2} - 2:\]
\[9 \cdot \left( t^{2} - 2 \right) - 18t = 22\]
\[9t^{2} - 18 - 18t - 22 = 0\]
\[9t^{2} - 18t - 40 = 0\]
\[D_{1} = 81 + 9 \cdot 40 = 441\]
\[t_{1,2} = \frac{9 \pm 21}{9} = - \frac{4}{3};\frac{10}{3}\text{.\ }\]
\[1)\ x + \frac{1}{x} = \frac{10}{3}\]
\[3x^{2} - 10x + 3 = 0\]
\[D = 25 - 9 = 16\]
\[x_{1,2} = \frac{5 \pm 4}{3} = 3;\frac{1}{3}\text{.\ }\]
\[2)\ x + \frac{1}{x} = - \frac{4}{3}\ \]
\[3x^{2} - 4x + 3 = 0\]
\[D = 4 - 3 \cdot 3 < 0 \Longrightarrow\]
\[\Longrightarrow корней\ нет.\]
\[Ответ:\ x = \frac{1}{3};\ \ x = 3.\]
