Решебник по алгебре 8 класс Мерзляк ФГОС Задание 543

\[\boxed{\mathbf{543\ (543).\ }Еуроки\ - \ ДЗ\ без\ мороки}\]

\[1)\frac{a}{\sqrt{11}} = \frac{a\sqrt{11}}{11};\]

\[2)\frac{18}{\sqrt{6}} = \frac{18\sqrt{6}}{6} = 3\sqrt{6};\]

\[3)\frac{5}{\sqrt{10}} = \frac{5\sqrt{10}}{10} = \frac{\sqrt{10}}{2};\]

\[4)\frac{13}{\sqrt{26}} = \frac{13\sqrt{26}}{26} = \frac{\sqrt{26}}{2};\]

\[5)\frac{30}{\sqrt{15}} = \frac{30\sqrt{15}}{15} = 2\sqrt{15};\]

\[6)\frac{2}{3\sqrt{x}} = \frac{2\sqrt{x}}{3x}\text{.\ }\]

\[\boxed{\mathbf{5}\mathbf{4}\mathbf{3}\mathbf{\text{.\ }}Еуроки\ - \ ДЗ\ без\ мороки}\]

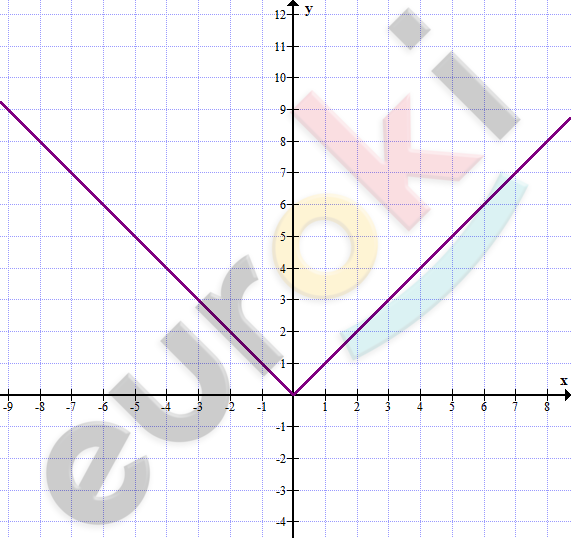
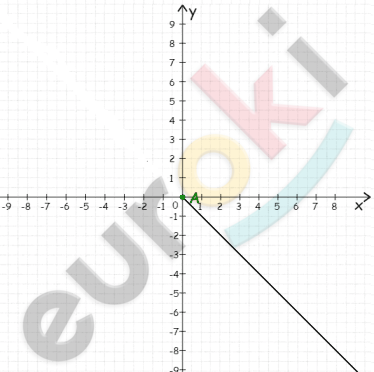
\[1)\ y = \sqrt{x^{2}} - 2x,\ \ x \geq 0\]

\[y = |x| - 2x\]

\[y = x - 2x\]

\[y = - x\]

\[2)\ y = \sqrt{- x} \cdot \sqrt{- x}\]

\[y = \sqrt{x^{2}}\]

\[y = |x|\]

\[y = \left\{ \begin{matrix} x,\ \ x \geq 0 \\ - x,\ \ x < 0 \\ \end{matrix} \right.\ \ \]