Решебник по алгебре 8 класс Мерзляк ФГОС Задание 337

\[\boxed{\text{337\ (337).}\text{\ }\text{Еуроки\ -\ ДЗ\ без\ мороки}}\]

\[так\ как\ x = y:\]

\[x = \frac{64}{x}\]

\[x^{\backslash x} - \frac{64}{x} = 0\]

\[\frac{x^{2} - 64}{x} = 0\]

\[\left\{ \begin{matrix} x^{2} - 64 = 0 \\ x \neq 0\ \ \ \ \ \ \ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ \ }\left\{ \begin{matrix} x = 8\ \ \ \\ x = - 8 \\ x \neq 0\ \ \ \\ \end{matrix} \right.\ \]

\[\text{A\ }(8;8);\ \ B\ ( - 8;\ - 8)\]

\[Ответ:\ A\ (8;8);\ \ B\ ( - 8;\ - 8).\]

\[\boxed{\text{337.}\text{\ }\text{Еуроки\ -\ ДЗ\ без\ мороки}}\]

\[y = \frac{k}{x}\]

\[\text{A\ }(10;1,6):\ \]

\[1,6 = \frac{k}{10}\text{\ \ }\]

\[k = 1,6 \cdot 10 = 16\]

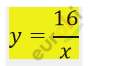
\[1)\ \text{B\ }( - 1;\ - 16):\ \ \]

\[- 16 = \frac{16}{- 1}\]

\[- 16 = - 16 \Longrightarrow проходит.\]

\[2)\ \text{C\ }( - 2;8):\ \ \]

\[8 = \frac{16}{- 2}\ \]

\[8 \neq - 8 \Longrightarrow \ не\ проходит.\]