Решебник по алгебре 8 класс Мерзляк ФГОС Задание 1042

\[\boxed{\mathbf{1042.\ }Еуроки\ - \ ДЗ\ без\ мороки}\]

\[1)\ \sqrt{8 - x} + \frac{1}{2\sqrt{x}}\]

\[\left\{ \begin{matrix} 8 - x \geq 0 \\ 2\sqrt{x} \neq 0\ \ \\ x > 0\ \ \ \ \ \ \ \\ \end{matrix}\ \right.\ \text{\ \ \ \ \ \ }\]

\[\left\{ \begin{matrix} - x \geq - 8 \\ \sqrt{x} \neq 0\ \ \ \\ x > 0\ \ \ \ \ \ \ \\ \end{matrix} \right.\ \text{\ \ \ \ \ }\]

\[\left\{ \begin{matrix} x \leq 8 \\ x \neq 0 \\ x > 0 \\ \end{matrix} \right.\ \]

\[Ответ:x \in (0;8\rbrack.\]

\[2)\ \sqrt{7x - 35} + \frac{1}{x^{2} - 5x}\]

\[\left\{ \begin{matrix} 7x - 35 \geq 0 \\ x^{2} - 5x \neq 0 \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ }\]

\[\left\{ \begin{matrix} 7x \geq 35\ \ \ \ \ \ \ \ \\ x(x - 5) \neq 0 \\ \end{matrix} \right.\ \text{\ \ }\]

\[\ \left\{ \begin{matrix} x \geq 5 \\ x \neq 0 \\ x \neq 5 \\ \end{matrix} \right.\ \]

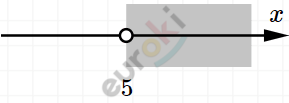
\[Ответ:x \in (5;\ + \infty).\]