Алгебра
Английский
Биология
География
Геометрия
Русский
История
Обществознание
Физика
Химия
Информатика
Литература
МатематикаГДЗ по алгебре 8 класс Мерзляк Проверь себя 3
Проверь себя 3
\[\boxed{\text{Задание}\text{\ \ 3.\ }\text{Еуроки\ -\ ДЗ\ без\ мороки}}\]
\[\boxed{\mathbf{1.}}\]
\[\frac{x^{2} - 100}{x - 10} = 0\]
\[\frac{(x - 10)(x + 10)}{x - 10} = 0\]
\[Ответ:В).\]
\[\boxed{\mathbf{2.}}\]
\[\frac{x - 10}{x^{2} - 100} = 0\]
\[\frac{x - 10}{(x - 10)(x + 10)} = 0\]
\[Ответ:Г).\]
\[\boxed{\mathbf{3.}}\]
\[А)\ 10^{- 3} = - 1000\]
\[0,001 \neq - 1000\]
\[Б)\ \left( - 1\frac{1}{3} \right)^{2} = - \frac{9}{16}\]
\[\left( - \frac{4}{3} \right)^{2} = - \frac{9}{16}\]
\[\frac{16}{9} \neq - \frac{9}{16}\]
\[В)\ ( - 2)^{- 3} = - \frac{1}{8}\]
\[( - 2)^{- 3} = - \frac{1}{2^{3}} = - \frac{1}{8}\]
\[Г)\ \frac{1}{7^{- 2}} = - 49\]
\[7^{2} = - 49\]
\[49 \neq - 49\]
\[Ответ:В).\]
\[\boxed{\mathbf{4.}}\]
\[42\ 000 = 4,2 \cdot 10^{4}\]
\[Ответ:Б).\]
\[\boxed{\mathbf{5.}}\]
\[6,3 \cdot 10^{- 3} = 0,0063\]
\[Ответ:В).\]
\[\boxed{\mathbf{6.}}\]
\[\frac{1}{25} = \frac{1}{5^{2}} = 5^{- 2}\]
\[Ответ:А).\]
\[\boxed{\mathbf{7.}}\]
\[\left( 1,7 \cdot 10^{8} \right) \cdot \left( 6 \cdot 10^{- 3} \right) =\]
\[= 1,7 \cdot 6 \cdot 10^{8} \cdot 10^{- 3} =\]
\[= 10,2 \cdot 10^{5} = 1,02 \cdot 10^{6}\]
\[Ответ:Б).\]
\[\boxed{\mathbf{8.}}\]
\[\frac{9^{- 2} \cdot 3^{- 5}}{81 \cdot 27^{- 3}} = \frac{\left( 3^{2} \right)^{- 2} \cdot 3^{- 5}}{3^{4} \cdot \left( 3^{3} \right)^{- 3}} =\]
\[= \frac{3^{- 4} \cdot 3^{- 5}}{3^{4} \cdot 3^{- 9}} = \frac{3^{- 9}}{3^{4} \cdot 3^{- 9}} = \frac{1}{3^{4}} =\]
\[= \frac{1}{81}\]
\[Ответ:Б.\]
\[\boxed{\mathbf{9.}}\]
\[y = \frac{3x}{2} = 1,5x - не\ является\ \]
\[графиком\ обратной\ \]
\[пропорциональности.\]
\[Ответ:Г.\]
\[\boxed{\mathbf{10.}}\]
\[y = - \frac{4}{x}\]
\[\mathbf{график\ функции\ расположен\ }\]
\[\mathbf{во\ второй\ и\ четвертой\ }\]
\[\mathbf{четвертях.}\]
\[Ответ:А).\]
\[\boxed{\mathbf{11.}}\]
\[y = \frac{k}{x}\]
\[\text{A\ }( - 3;0,6):\]
\[- 3 = \frac{k}{0,6}\]
\[k = - 3 \cdot 0,6 = - 1,8\]
\[Ответ:А).\]
\[\boxed{\mathbf{12.}}\]
\[\frac{2x - 1}{x + 4} - \frac{3x + 1}{4 - x} = \frac{4x^{2} + 8}{x^{2} - 16}\]
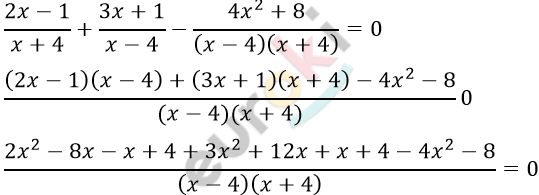
\[\frac{x^{2} + 4x}{(x - 4)(x + 4)} = 0\]
\[\frac{x(x + 4)}{(x - 4)(x + 4)} = 0\]
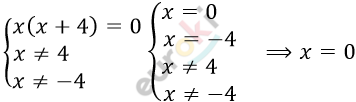
\[Ответ:Г).\]
