Решебник по алгебре и начала математического анализа 11 класс Колягин Задание 396

\[1)\ y = x^{2} - 6x + 9;\]

\[y = x^{2} + 4x + 4;\ \ \text{\ y} = 0:\]

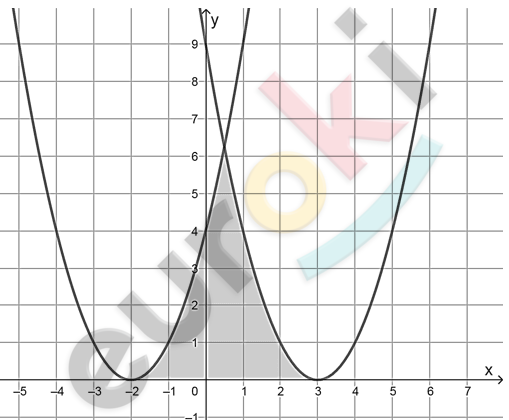
\[= \int_{\frac{1}{2}}^{3}{(x - 3)^{2}\text{dx}} + \int_{- 2}^{\frac{1}{2}}{(x + 2)^{2}\text{dx}} =\]

\[= \left. \ \frac{(x - 3)^{3}}{3} \right|_{\frac{1}{2}}^{3} + \left. \ \frac{(x + 2)^{3}}{3} \right|_{- 2}^{\frac{1}{2}} =\]

\[= \frac{0^{3}}{3} - \frac{1}{3} \bullet \left( - \frac{5}{2} \right)^{3} + \frac{1}{3} \bullet \left( \frac{5}{2} \right)^{3} - \frac{0^{3}}{3} =\]

\[= \frac{2}{3} \bullet \left( \frac{5}{2} \right)^{3} = \frac{250}{24} = \frac{125}{12} = 10\frac{5}{12}.\]

\[Ответ:\ \ 10\frac{5}{12}.\]

\[2)\ y = x^{2} + 1;\ \ \ y = 3 - x^{2}:\]

\[x^{2} + 1 = 3 - x^{2}\]

\[2x^{2} = 2\]

\[x^{2} = 1\]

\[x = \pm 1.\]

\[|S| = \int_{- 1}^{1}{\left( x^{2} + 1 - 3 + x^{2} \right)\text{dx}} =\]

\[= \int_{- 1}^{1}{\left( 2x^{2} - 2 \right)\text{dx}} =\]

\[= \left. \ \left( \frac{2}{3}x^{3} - 2x \right) \right|_{- 1}^{1} =\]

\[= \left( \frac{2}{3} - 2 \right) - \left( - \frac{2}{3} + 2 \right) = \frac{4}{3} - 4 =\]

\[= 1\frac{1}{3} - 4 = - 2\frac{2}{3}.\]

\[Ответ:\ \ 2\frac{2}{3}.\]

\[3)\ y = x^{2};\ \ \ y = 2\sqrt{2x}:\]

\[x^{2} = 2\sqrt{2x}\]

\[x^{4} = 4 \bullet 2x\]

\[x\left( x^{3} - 8 \right) = 0\]

\[x_{1} = 0;\text{\ \ \ }x_{2} = 2.\]

\[|S| = \int_{0}^{2}{\left( x^{2} - 2\sqrt{2x} \right)\text{dx}} =\]

\[= \left. \ \left( \frac{x^{3}}{3} - 2 \bullet \frac{1}{2} \bullet (2x)^{\frac{3}{2}}\ :\frac{3}{2} \right) \right|_{0}^{2} =\]

\[= \left. \ \left( \frac{x^{3}}{3} - \frac{2}{3}(2x)^{\frac{3}{2}} \right) \right|_{0}^{2} =\]

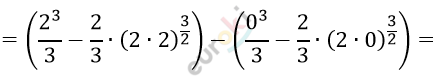
\[= \frac{8}{3} - \frac{2}{3} \bullet 2^{3} = - \frac{8}{3} = - 2\frac{2}{3}.\]

\[Ответ:\ \ 2\frac{2}{3}.\]

\[4)\ y = \sqrt{x};\ y = \sqrt{4 - 3x};\text{\ y} = 0:\]

\[S = \int_{1}^{\frac{4}{3}}{\sqrt{4 - 3x}\text{\ dx}} + \int_{0}^{1}{\sqrt{x}\text{\ dx}} =\]

\[= \left. \ \left( - \frac{1}{3}(4 - 3x)^{\frac{3}{2}}\ :\frac{3}{2} \right) \right|_{1}^{\frac{4}{3}} + \left. \ \left( x^{\frac{3}{2}}\ :\frac{3}{2} \right) \right|_{0}^{1} =\]

\[= \left. \ - \frac{2}{9}(4 - 3x)^{\frac{3}{2}} \right|_{1}^{\frac{4}{3}} + \left. \ \frac{2}{3}x^{\frac{3}{2}} \right|_{0}^{1} =\]

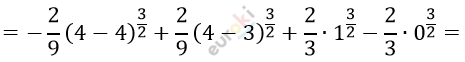
\[= - \frac{2}{9} \bullet 0^{\frac{3}{2}} + \frac{2}{9} \bullet 1^{\frac{3}{2}} + \frac{2}{3} \bullet 1 - 0 =\]

\[= \frac{2}{9} + \frac{2}{3} = \frac{2}{9} + \frac{6}{9} = \frac{8}{9}.\]

\[Ответ:\ \ \frac{8}{9}.\]