Решебник по алгебре и начала математического анализа 11 класс Алимов Задание 1608

\[\boxed{\mathbf{1608}\mathbf{.}}\]

\[\lg(y - 4x) = \lg\frac{(2 + 2x - y)^{2}}{y}\]

\[y - 4x = \frac{(2 + 2x - y)^{2}}{y}\ \ \ \ \ | \bullet y\]

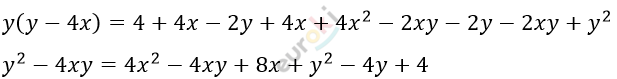
\[0 = 4x^{2} + 8x - 4y + 4\]

\[x^{2} + 2x - y + 1 = 0\]

\[y = x^{2} + 2x + 1\]

\[Подставим\ y:\]

\[27 \bullet 3^{2x - \left( x^{2} + 2x + 1 \right)} + 3^{x^{2}} = 4\sqrt{3}\]

\[3^{3} \bullet 3^{- x^{2} - 1} + 3^{x^{2}} = 4\sqrt{3}\]

\[3^{2 - x^{2}} + 3^{x^{2}} - 4\sqrt{3} = 0\]

\[z = 3^{x^{2}}:\]

\[\frac{3^{2}}{z} + z - 4\sqrt{3} = 0\ \ \ \ \ | \bullet z\]

\[z^{2} - 4\sqrt{3}z + 9 = 0\]

\[D = 48 - 36 = 12 = 4 \bullet 3\]

\[z_{1} = \frac{4\sqrt{3} - \sqrt{12}}{2} = \frac{4\sqrt{3} - 2\sqrt{3}}{2} =\]

\[= \frac{2\sqrt{3}}{2} = \sqrt{3} = 3^{\frac{1}{2}};\]

\[z_{2} = \frac{4\sqrt{3} + \sqrt{12}}{2} = \frac{4\sqrt{3} + 2\sqrt{3}}{2} =\]

\[= \frac{6\sqrt{3}}{2} = 3\sqrt{3} = 3^{\frac{3}{2}}.\]

\[1)\ 3^{x^{2}} = 3^{\frac{1}{2}}\]

\[x^{2} = \frac{1}{2}\]

\[x = \pm \frac{1}{\sqrt{2}};\]

\[y_{1} = \frac{1}{2} + \frac{2}{\sqrt{2}} + 1 = \frac{3}{2} + \sqrt{2};\]

\[y_{2} = \frac{1}{2} - \frac{2}{\sqrt{2}} + 1 = \frac{3}{2} - \sqrt{2}.\]

\[2)\ 3^{x^{2}} = 3^{\frac{3}{2}}\]

\[x^{2} = \frac{3}{2}\]

\[\ x = \pm \sqrt{\frac{3}{2}};\]

\[y_{1} = \frac{3}{2} + 2\sqrt{\frac{3}{2}} + 1 =\]

\[= \frac{5}{2} + \sqrt{2 \bullet 3} = \frac{5}{2} + \sqrt{6};\]

\[y_{2} = \frac{3}{2} - 2\sqrt{\frac{3}{2}} + 1 =\]

\[= \frac{5}{2} - \sqrt{2 \bullet 3} = \frac{5}{2} - \sqrt{6}.\]

\[Имеет\ смысл\ при:\]

\[y - 4x > 0\]

\[y > 4x;\]

\[2 + 2x - y > 0\]

\[y < 2 + 2x;\]

\[y > 0.\]

\[Ответ:\ \ \left( \frac{1}{\sqrt{2}};\ \frac{3}{2} + \sqrt{2} \right);\ \ \]

\[\text{\ \ \ \ \ \ \ \ \ \ \ \ \ }\left( - \frac{1}{\sqrt{2}};\ \frac{3}{2} - \sqrt{2} \right).\]

\[\lg(x + 4y) = \lg(2 - x - 2y)^{2} - \lg x\]

\[\lg(x + 4y) = \lg\frac{(2 - x - 2y)^{2}}{x}\]

\[x + 4y = \frac{(2 - x - 2y)^{2}}{x}\ \ \ \ \ | \bullet x\]

\[0 = 4y^{2} - 4x - 8y + 4\]

\[y^{2} - x - 2y + 1 = 0\]

\[x = y^{2} - 2y + 1\]

\[Подставим:\]

\[8 \bullet 2^{- \left( y^{2} - 2y + 1 \right) - 2y} + 2^{y^{2}} = 3\sqrt{2}\]

\[2^{3} \bullet 2^{- y^{2} - 1} + 2^{y^{2}} = 3\sqrt{2}\]

\[2^{2 - y^{2}} + 2^{y^{2}} - 3\sqrt{2} = 0\]

\[z = 2^{y^{2}}:\]

\[\frac{2^{2}}{z} + z - 3\sqrt{2} = 0\ \ \ \ \ | \bullet z\]

\[z^{2} - 3\sqrt{2}z + 4 = 0\]

\[D = 18 - 16 = 2\]

\[z_{1} = \frac{3\sqrt{2} - \sqrt{2}}{2} = \frac{2\sqrt{2}}{2} =\]

\[= \sqrt{2} = 2^{\frac{1}{2}};\]

\[z_{2} = \frac{3\sqrt{2} + \sqrt{2}}{2} = \frac{4\sqrt{2}}{2} =\]

\[= 2\sqrt{2} = 2^{\frac{3}{2}};\]

\[1)\ 2^{y^{2}} = 2^{\frac{1}{2}}\]

\[y^{2} = \frac{1}{2}\]

\[y = \pm \frac{1}{\sqrt{2}};\]

\[x_{1} = \frac{1}{2} - \frac{2}{\sqrt{2}} + 1 = \frac{3}{2} - \sqrt{2};\]

\[x_{2} = \frac{1}{2} + \frac{2}{\sqrt{2}} + 1 = \frac{3}{2} + \sqrt{2}.\]

\[2)\ 2^{y^{2}} = 2^{\frac{3}{2}}\]

\[y^{2} = \frac{3}{2}\]

\[y = \pm \sqrt{\frac{3}{2}};\]

\[x_{1} = \frac{3}{2} - 2\sqrt{\frac{3}{2}} + 1 =\]

\[= \frac{5}{2} - \sqrt{2 \bullet 3} = \frac{5}{2} - \sqrt{6};\]

\[x_{2} = \frac{3}{2} + 2\sqrt{\frac{3}{2}} + 1 =\]

\[= \frac{5}{2} + \sqrt{2 \bullet 3} = \frac{5}{2} + \sqrt{6}.\]

\[Имеет\ смысл\ при:\]

\[x + 4y > 0 \rightarrow x > - 4y;\]

\[2 - x - 2y > 0 \rightarrow x < 2 - 2y;\]

\[x > 0.\]

\[Ответ:\ \ \left( \frac{3}{2} - \sqrt{2};\ \frac{1}{\sqrt{2}} \right);\ \ \]

\[\text{\ \ \ \ \ \ \ \ \ \ \ \ }\left( \frac{3}{2} + \sqrt{2};\ - \frac{1}{\sqrt{2}} \right).\]