Решебник по алгебре и начала математического анализа 11 класс Алимов Задание 1008

\[\boxed{\mathbf{1008}\mathbf{.}}\]

\[1)\int_{- 2}^{1}{x(x + 3)(2x - 1)\text{\ dx}} =\]

\[= \int_{- 2}^{1}{\left( 2x^{3} - x^{2} + 6x^{2} - 3x \right)\text{\ dx}} =\]

\[= \int_{- 2}^{1}{\left( 2x^{3} + 5x^{2} - 3x \right)\text{\ dx}} =\]

\[= \left. \ \left( 2 \bullet \frac{x^{4}}{4} + 5 \bullet \frac{x^{3}}{3} - 3 \bullet \frac{x^{2}}{2} \right) \right|_{- 2}^{1} =\]

\[= \left. \ \left( \frac{x^{4}}{2} + 5 \bullet \frac{x^{3}}{3} - 3 \bullet \frac{x^{2}}{2} \right) \right|_{- 2}^{1} =\]

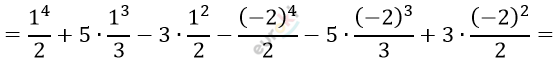
\[= \frac{1}{2} + \frac{5}{3} - \frac{3}{2} - \frac{16}{2} + \frac{40}{3} + \frac{12}{2} =\]

\[= - \frac{6}{2} + \frac{45}{3} = - 3 + 15 = 12;\]

\[2)\int_{- 1}^{0}{(x + 1)\left( x^{2} - 2 \right)\text{\ dx}} =\]

\[= \int_{- 1}^{0}{\left( x^{3} - 2x + x^{2} - 2 \right)\text{\ dx}} =\]

\[= - \frac{1}{4} + 1 + \frac{1}{3} - 2 =\]

\[= - 1 - \frac{3}{12} + \frac{4}{12} = - 1 + \frac{1}{12} =\]

\[= - \frac{11}{12};\]

\[3)\int_{1}^{2}{\left( x + \frac{1}{x} \right)^{2}\text{\ dx}} =\]

\[= \int_{1}^{2}{\left( x^{2} + 2 \bullet x \bullet \frac{1}{x} + \frac{1}{x^{2}} \right)\text{\ dx}} =\]

\[= \int_{1}^{2}{\left( x^{2} + 2 + x^{- 2} \right)\text{\ dx}} =\]

\[= \left. \ \left( \frac{x^{3}}{3} + 2 \bullet \frac{x^{1}}{1} + \frac{x^{- 1}}{- 1} \right) \right|_{1}^{2} =\]

\[= \left. \ \left( \frac{x^{3}}{3} + 2x - \frac{1}{x} \right) \right|_{1}^{2} =\]

\[= \frac{2^{3}}{3} + 2 \bullet 2 - \frac{1}{2} - \frac{1^{3}}{3} - 2 + 1 =\]

\[= \frac{8}{3} + 4 - \frac{1}{2} - \frac{1}{3} - 1 =\]

\[= 3 + \frac{7}{3} - \frac{1}{2} = 3 + \frac{14}{6} - \frac{3}{6} =\]

\[= 3 + \frac{11}{6} = 3 + 1\frac{5}{6} = 4\frac{5}{6};\]

\[4)\int_{- 2}^{- 1}{\frac{4}{x^{2}}\left( 1 - \frac{2}{x} \right)\text{\ dx}} =\]

\[= \int_{- 2}^{- 1}{\left( \frac{4}{x^{2}} - \frac{8}{x^{3}} \right)\text{\ dx}} =\]

\[= \int_{- 2}^{- 1}{\left( 4 \bullet x^{- 2} - 8 \bullet x^{- 3} \right)\text{\ dx}} =\]

\[= \left. \ \left( 4 \bullet \frac{x^{- 1}}{- 1} - 8 \bullet \frac{x^{- 2}}{- 2} \right) \right|_{- 2}^{- 1} =\]

\[= \left. \ \left( - \frac{4}{x} + \frac{4}{x^{2}} \right) \right|_{- 2}^{- 1} =\]

\[= - \frac{4}{- 1} + \frac{4}{( - 1)^{2}} + \frac{4}{- 2} - \frac{4}{( - 2)^{2}} =\]

\[= 4 + 4 - 2 - 1 = 5.\]