Найдите корни уравнения: 1/(x-2)^2 +9/(x+2)^2 -6/(x^2-4)=0.

Ответ:

\[\frac{1}{(x - 2)^{2}} + \frac{9}{(x + 2)^{2}} - \frac{6}{x^{2} - 4} = 0\]

\[ОДЗ:x - 2 \neq 0 \Longrightarrow x \neq 2\]

\[\ \ \ \ \ \ x + 2 \neq 0 \Longrightarrow x \neq - 2\]

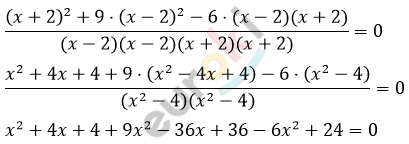
\[4x² - 32x + 64 = 0\ \ \ \ \ \ \ \ |\ :4\]

\[x^{2} - 8x + 16 = 0\]

\[x_{1} + x_{2} = 8\]

\[x_{1} \cdot x_{2} = 16 \Longrightarrow x_{1} = 4;\ \ x_{2} = 4\]

\[Ответ:x = 4.\]